Maximum Implied Variance Slope
The paper The Moment Formula for Implied Volatility at Extreme Strikes by Roger Lee redefined how practioners extrapolate the implied volatility, by showing that the total implied variance can be at most linear in the wings, with a slope below 2.
Shortly after, the SVI model of Jim Gatheral, with its linear wings, started to become popular.
In a recent paper in collaboration with Winfried Koller, we show that the asymptotic bounds are usually overly optimistic. This is somewhat expected, as the bound only holds as the log-moneyness goes to infinity. What is less expected, is that, even at very large strikes (nearly up to the limit of what SVI allow numerically), we may happen to have arbitrages, even though the bounds are respected.
In practice, this manifests by increasing call option prices far away in the wings (increasing call prices are an arbitrage opportunity), which could be problematic for any kind of replication based pricing, such as variance swap pricing.
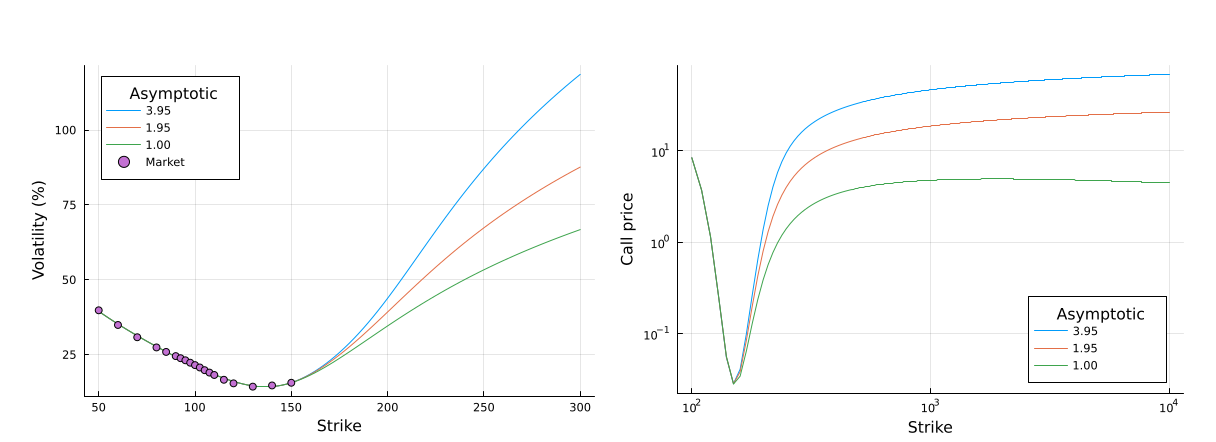
Increasing call prices for various calibrated SVI parameters respecting the asymptotic boundary constraints.
The paper on arxiv.